Updated July 24, 2023
Gamma Function Formula (Table of Contents)
What is the Gamma Function Formula?
People will be aware of the three Greek symbols Alpha, Beta, and Gamma from the secondary school itself in the mathematics world. These are predominantly used in physics and widely used in Algebraic mathematical functions and integral functions.
People often recognize the gamma function as a well-known factorial symbol. It was hosted by the famous mathematician L. Euler (Swiss Mathematician 1707 – 1783) as a natural extension of the factorial operation from positive integers to real and even complex values of an argument. This Gamma function is calculated using the following formulae:
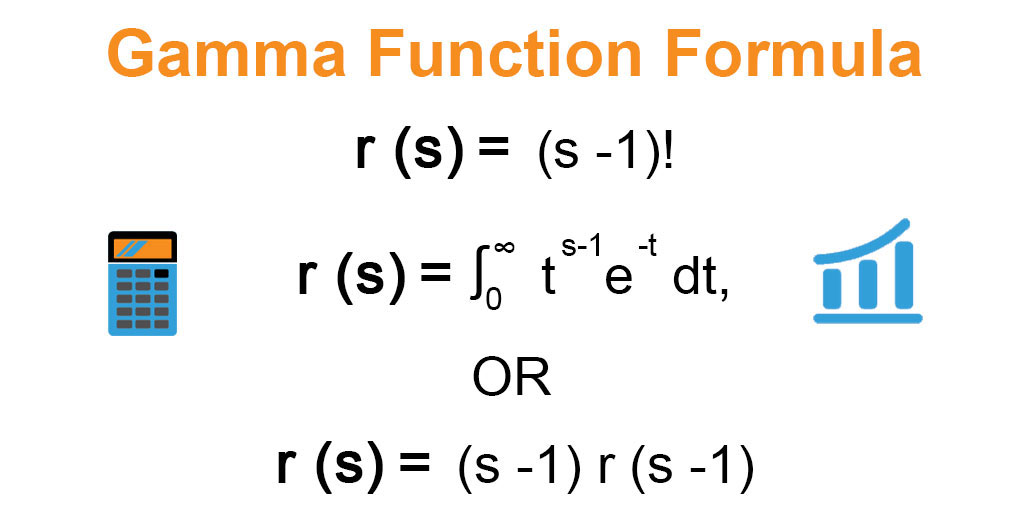
To identify the Gamma function.
Where,
s: Positive Integer
To identify the Gamma function for other integers:
This can be further simplified as follows:
Where,
s: positive real number, and s should always be greater than 0.
Example of Gamma Function Formula
Let’s take an example to understand the calculation of the Gamma Function in a better manner.
Gamma Function Formula – Example #1
If the number is a ‘s’ and is a positive integer, then the gamma function will be the factorial of the number. This is mentioned as s! = 1*2*3… (s − 1)*s. For example, 4! = 1 × 2 × 3 × 4 = 24. However, this formula is not valid if s is not an integer.
Solution:
Γ(4) = (4-1)! = 6
Gamma Function Formula – Example #2
Evaluate Gamma Function Value for Γ (3/2) / Γ (5/2)
Solution:
Step 1: Identify whether the number is an integer. In this case, it is not an integer.
Step 2: Apply the simplified version of the second formula:
Γ (5/2) = (s-1) Γ (s-1)
- Γ (5/2) = ((5/2)-1) Γ ((5/2)-1)
- Γ (5/2) = (3/2) Γ (3/2)
Step 3: Now apply the value for (5/2) in the original equation
- Γ (3/2) / Γ (5/2)
- = Γ (3/2) / (3/2) Γ (3/2)
Step 4: Cancelling Γ (3/2) as it is part of both numerator and denominator, the final value will be (3/2)
Hence the conclusion for the equation will be Γ (3/2) / Γ (5/2) = (3/2)
Explanation
The formula for Gamma Function Formula can be calculated by using the following steps:
Step 1: Identify whether the input value is an integer or a real number.
Step 2: If it is an integer, we must use 1st formulae, i.e., identifying the integer value’s factorial – 1.
In the mathematics world, the word “Factorial” denotes the product (multiplication) of positive integers, which is < or = the input integer. That integer always denotes it, followed by an exclamatory note. Thus, any factorial (s) is written S!, and if s = 4 meaning, it is 4! Which is equal to 1 × 2 × 3 × 4. Always note that zero factorial is always equal to 1!!
Step 3: If it is a positive real number, we must use the 2nd formula. For example, we need to identify the Γ value for the number (5/2). Applying to the simplified formulae, it will be (s-1) Γ (s-1). In this case, (5/2)-1 Γ (5/2)-1 which will be further simplified as (3/2) Γ (3/2)
Relevance and Use of Gamma Function Formula
The integral in Γ(1) is convergent, which can be proved easily. There are some unique properties of the gamma function. Below are the properties specific to the gamma function:
It is proved that:
- Γ(s + 1) = sΓ(s), since
- Γ(s + 1) = lim T→∞ (Integral of 0 → T) e −t tp dt
- = p (Integral 0 → ∞) e −t tp-1 dt
- = pΓ(p)
- Γ(1) = 1 (inconsequential proof)
- If s = n, a positive integer, then Γ(n + 1) = n!
The gamma function is applicable for all complex numbers (A complex number is defined as a number in common. It has two parts. The first one is a real number, followed by an imaginary number. The common imaginary number used in mathematics is the small letter “i,” and the square of i is always equal to the constant -1). Negative integers and zero do not have definitions for them. We consider all natural numbers as a discrete set and all positive real numbers as a continuous set. Please note that the Gamma function will behave like a normal factorial for all natural numbers. However, the leeway to the real positive numbers makes it helpful in modeling sites that include continuous change, with vital applications to differential and integral calculus, differential equations, complex analysis, and statistics.
Recommended Articles
This is a guide to Gamma Function Formula. Here we discuss how to calculate the Gamma Function? Along with practical examples. You may also look at the following articles to learn more –